Engr Help

Composite Beams

Key Concepts: A composite beam is one where two or more sections of the beam,

with differing materials (modulus of Elasticity, E), are “laminated” together to form a

composite beam. The strategy for analysis of composite beams is to convert the

laminated sections to one material recognizing that materials resist bending in direct

proportion to their modulus of elasticity, E. Then apply the usual formula for bending

stress, σ = Mc/I .

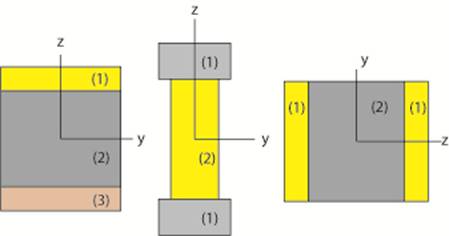
In a Nut Shell: The use of composite beams may result from cost or strength

considerations. The figure below shows three different x-sections of a composite beam.

The beam on the top left has three different materials with moduli of elasticity, E₁, E₂,

and E₃. The beam in the middle is an I-beam with two materials symmetrically placed.

Likewise the beam on the riight has two materials symmetrically placed.

Procedure for Analysis of Composite Beams:

Pick one of the materials to be the “base material”. i.e. material one. E₁

Form a new transformed section with one material by multiplying the widths of the

x-section by E₂/E₁ etc Call it the “transformed x-section”.

Calculate the moment of inertia of the entire, transformed x-section, I

Use it to calculate the stress at desired locations by using σ = Mc/I where I

is about the neutral axis of the reformed x-section.

Multiply the stress calculated in step 3 by E₁/E₂ to obtain the stresses in material 2.

Return to Notes on Solid Mechanics